The Bernoulli equation is one of the fundamental principles in fluid mechanics, used to describe the flow of incompressible fluids. It applies the principle of energy conservation to the flow of a fluid, representing the relationship among the fluid's velocity. pressure, and potential energy due to its position.
The Bernoulli equation is derived under the ideal conditions of steady flow, incompressibility, and no friction, making its application to real-world situations challenging. The core idea of the Bernoulli equation is that as a fluid flows, an increase in the fluid's velosity lead to a decrease in pressure, and conversely, a decrease in velocity results in an increase in pressure.
From the general momentum equation, assuming the existence of an ideal fluid allows fow the transition to the Euler equations.
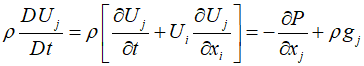
By multiplying with ${U_j}$, the mechanical energy equation for frictionless fluid flow can be derived.
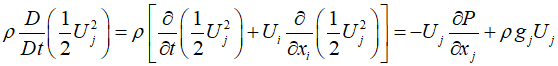
Where ${U_j}$ represent the fluid velocity in the $j$ direction, and $D{U_j}/Dt$ is the material derivative of ${U_j}$, representing the rate of change of a quantity with respect to time for fluid particle.
${G_j}$ can be represented as follows.
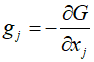
Where $G$ represents the gravitational potential, indicating the potential energy due to gravity for a unit mass at a point. Substituting this into the mechanical energy equation yields the following.
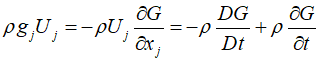
The equation can be derived using tensor notation, and since $\partial G/\partial t = 0$, it follows that.
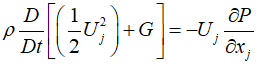
Through the above equations, the mechanical energy equation for frictionless fluid flow has been derived, demonstraing that the sum of the fluid's kinetic energy and gravitational potential energy changes due to the action caused by pressure variations.
Including the ratio of pressure to density changes, a more general form of the Bernoulli equation can be derived. The rate of change of this ratio for a fluid particle over time is represented as follows.
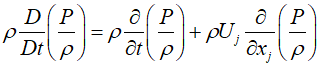
Using the rules of differentiation, the above equation can be simplified as follows.
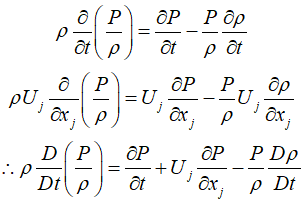
By combining the rate of change of $P/\rho $ wth tha fluid's mechanical energy equation, the rate of change of the fluid's total energy can be represented.
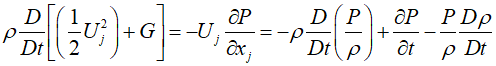
Alternatively, it can be represented as follows.

From the above equation, assuming there is no change in pressure in steady state $\partial P/\partial t = 0$ and the density is constant, the following Bernoulli equation can be obtained.

'Fluid Dynamics' 카테고리의 다른 글
| 90_Momentum Equation (0) | 2024.02.28 |
|---|---|
| 89_Continuity Equation (0) | 2024.02.27 |
| 87_Equation of Motion (0) | 2024.02.25 |
| 86_Newton's Second Law of Motion (0) | 2024.02.24 |
| 85_Hyrdostatics (0) | 2024.02.23 |