The Cauchy momentum equation is a partial differential equation presented by Cauchy that describes the transport of momentum in all continua, and it can be represented as follows.
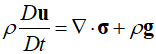
Using the point particle accerlation ${\bf{a}}$ and the hydrodynamic volume force $\sum \equiv \nabla \cdot {\bf{\sigma }}$ in the Cauchy momentum equation simplifies it as follows.
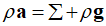
Here, through the vector form of fluid velocity, the equation can be modified as follows.
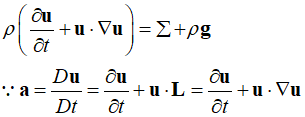
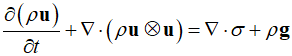
For each of the $x, y, z$ components, it can be represented as follows.
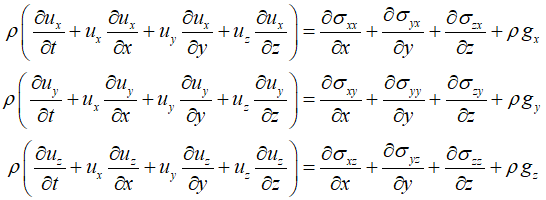
The terms inside the parentheses on the left side represent the Cartesian components of the point paricle acceleration, and on the right side, they represent the Cartesian components of the volume forces due to fluid dynamic stress and the components of body forces.
The components of the momentum equation in vylindrical polar coordinates are as follows.
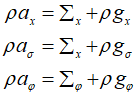
Using the cylindrical polar coordinate components ${a_x}$, ${a_\sigma }$, and ${a_\varphi }$, it can be modified as follows.
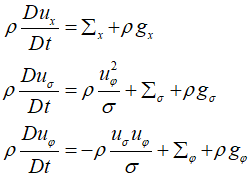
Here, the first term on the right side of the second equation, $\rho u_\varphi ^2/\sigma $, represents the effective volume force in the radial ($\sigma $) direction, which is the centrifugal force occurring as the rotating body spins around its own axis. The first term on the right side of the third equation, $ - \rho {u_\sigma }{u_\varphi }/\sigma $, represents the effective force in the radial direction, which occurs when the flow happens simultaneously in the radial and azimuthal ($\varphi $) directions, commonly known as the Coriolis force.
When representing the hydrodynamic volume force in spherical polar coordinates, it is as follows.
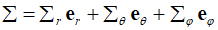
Using coordinate transformation and the chain rule, the spherical polar components can be represented as follows.
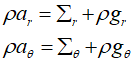
In summary, the flow of incompressible or compressible fluids is determined by the continuity equation, and through the three components of the motion equation combined with the continuity equation, a total of four equations can be obtained. For incompressible fluids, a fifth equation can be added due to the ideal incompressible condition. For compressible fluids, the fifth equation, which provides the relationship between density and pressure, can be obtained from thermodynamics.
'Fluid Dynamics' 카테고리의 다른 글
| 89_Continuity Equation (0) | 2024.02.27 |
|---|---|
| 88_Bernoulli Equation (1) | 2024.02.26 |
| 86_Newton's Second Law of Motion (0) | 2024.02.24 |
| 85_Hyrdostatics (0) | 2024.02.23 |
| 84_Energy Equation (0) | 2024.02.22 |