Hyrodstatics deal with the physics of fluids at rest, meaning fluids that are not in motion. It covers how fluids transmit forces and pressure, and how they affect the surrounding environment structures. Primarily, it involves analyzing the static states of fluids like water or air to study properties such as pressure, buoyancy, density, and their interactions within the fluid.
For an ideal gas, the density($\rho $) can be expressed in relation to pressure($p$) and temperature($T$) by the state equation as follows.
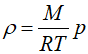
Where $M$ represents the molecular mass, and $R$ is the ideal-gas constant. By modifying the above equation, a vector equation that includes pressure and temperature can be derived.
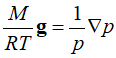
The $x$-component of the above equation is as follows.
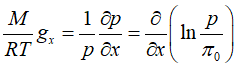
Where ${\pi _0}$ is represents an unspecified reference pressure. If the temperature of the fluid is uniform, integrating with respect to $x$ yields the following result.
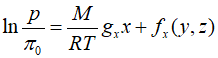
Where ${f_x}(y,z)$ is an unknown function, and since the temperature is uniform, a similar process can be applied for the $y$ and $z$ components as well.
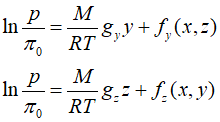
Similarly, ${f_y}(x,z)$ and ${f_z}(x,y)$ are two unknown functions, and by combining the three equations, the pressure distribution can be obtained.

Where ${\pi _0}$ is determined through appropriate boundary conditions. In the equation above, by expressing the terms inside the parentheses as the dot product of the gravity vector and the position vector, and mocing the last term to the left side, the pressure distribution for an ideal gas with uniform temperature can be succinctly represented.
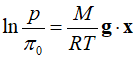
Additionally, liquids at low and moderate pressures are nearly incompressible, thus their density is represented as a physical property determined by the given temperature. Similar to the case with gases, but treating density as a constant, the pressure distribution is represented as follows.
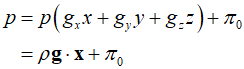
'Fluid Dynamics' 카테고리의 다른 글
| 88_Bernoulli Equation (1) | 2024.02.26 |
|---|---|
| 87_Equation of Motion (0) | 2024.02.25 |
| 86_Newton's Second Law of Motion (0) | 2024.02.24 |
| 84_Energy Equation (0) | 2024.02.22 |
| 83_Reynolds' Transport Theorem (0) | 2024.02.21 |