While conducting CFD analysis through Ansys Fluent, I realized my lack of theoretical knowledge in fluid dynamics. Therefore, I plan to study fluid mechanics theoretically. Since I haven't studied the theoretical aspects of fluid mechanics since my undergraduate courses, there might be inaccuracies, but I intend to write based on my understanding.
The first is the Reynold's Transport Theorem. The Reynolds Transport Theorem was developed to determine the rate of change over time of the physical properties of a fluid within a control volume. In other works, the Reynolds Transport Theorem is used to calculate the rate of change of momentum, and through this, to calculate forces.
Firstly, in three dimensions, the Leibniz rule is as follows.
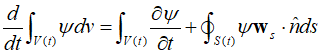
Where the volume integral on the right represents the change of the stream function($\psi $) within the volume $V$, and the surface integral calculates the transport of the stream function through the moving surface $S$.
If assume that $S(t)$ move together with the fluid velocity(${{\bf{w}}_s} = {\bf{w}}$), the fluid particles initially inside $V$ remain inside, and those outside remain outside. Therefore, $V(t)$ contains a fixed amount of mass, known as a material volume, similar to a closed thermodynamic system.
Consequently, the Leibniz rule can be modified as follows.
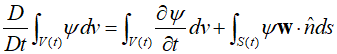
$\psi ({\bf{r}},t)$ can be a scaler, vector, or a higher-order tensor, and can be physically interpreted in the same way as the right-hand side of the previous Leibniz rule. If we do not assume that it moves with the fluid velocity( ${{\bf{w}}_s}=0$), then the volume and boundary surface are referred to as a fixed control volume(CV) and control surface(CS), respectively.
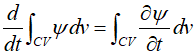
Through the above equation, the following relationship can be derived.
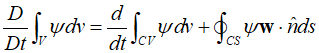
Where the left side represents the moving material volume, and the right-hand terms represent a fixed control volume, If $\psi $ is a scaler, the surface integral is as follows.
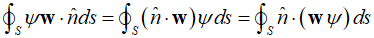
Through the divergence theorem, it can be summarized as follows.
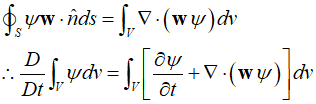
Divergence term can be expanded
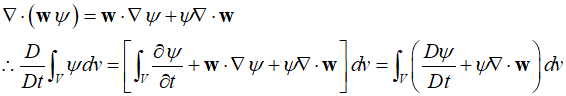
Additionally, for instance, if $\psi $ is 1 and $V$ is replaced with a small volume $\delta v$, the following equation can be obtained.
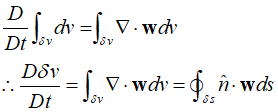
Once again, use the divergence theorem.
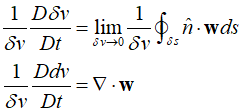
In the above equation, the left side represents the rate of volumetric strain of fluid particles, and through this equation, one can obtain the same equation as previously derived.

'Fluid Dynamics' 카테고리의 다른 글
| 88_Bernoulli Equation (1) | 2024.02.26 |
|---|---|
| 87_Equation of Motion (0) | 2024.02.25 |
| 86_Newton's Second Law of Motion (0) | 2024.02.24 |
| 85_Hyrdostatics (0) | 2024.02.23 |
| 84_Energy Equation (0) | 2024.02.22 |