The trapezoidal rule is the first formula in the Newton-Cotes integration formulas, and it corresponds to the case where the polynomial in the equation below is of the first degree.
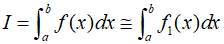
Here, the straight line can be expressed as follows.

The area under this straight line is an estimate of the integral between the interval $a$ and $b$.
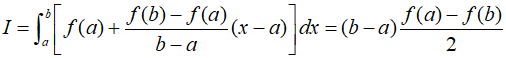
This is called the trapezoidal rule. The derivation process can be explained as follows.
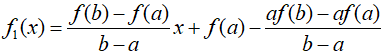
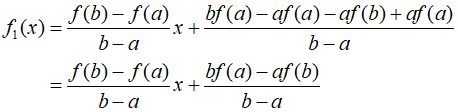
If we integrate this from $x=a$ to $x=b$, the calculation is as follows.
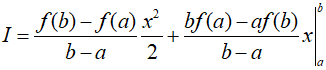
This can be simplified as follows.

Simplifying by setting ${b^2} - {a^2} = (b - a)(b + a)$, we get the following.

'Numerical Methods' 카테고리의 다른 글
| 158_Simpson's Rules (0) | 2024.05.06 |
|---|---|
| 157_Multiple Trapezoidal Rule (0) | 2024.05.05 |
| 155_Least Squares Fit (0) | 2024.05.03 |
| 154_Cubic Splines (0) | 2024.05.02 |
| 153_Quadratic Splines (0) | 2024.05.01 |